TRIG - Right Triangles and Trig

TRIG - Right Triangles and Trig

Mix of Circle problems

BELOW
I have given VERY STRONG HINTS

to all the ONES that I feel MOST STUDENTS

will be able to do.

The few that I did not do

are going to be difficult for everyone.

If you need explanations on

the ONES BELOW

we could talk on the phone SUNDAY.

GOOD LUCK...

*******************************************************

***********************************************************

***************************************************************

***************************************************************

****************************************

*****************************************

*************************************************

**************************************************************

************************************************************

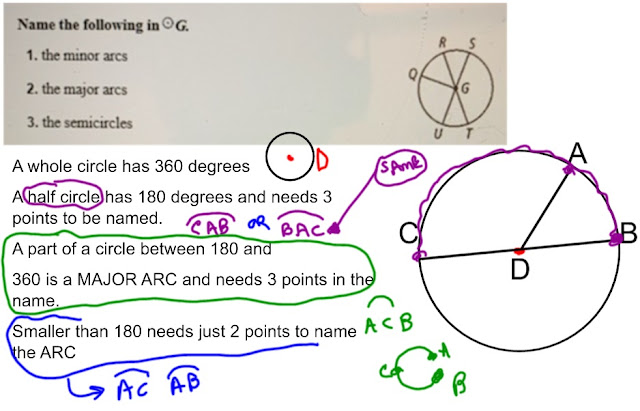
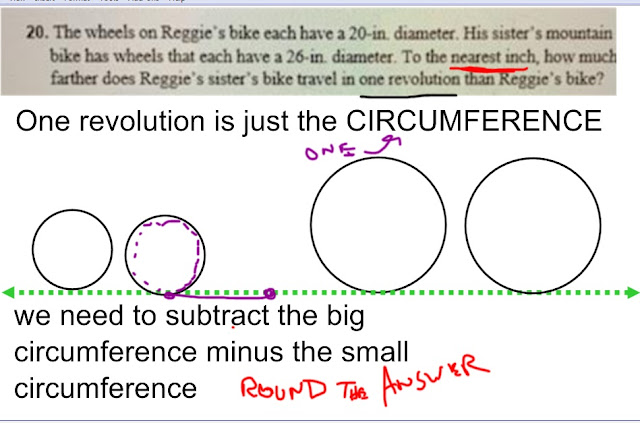
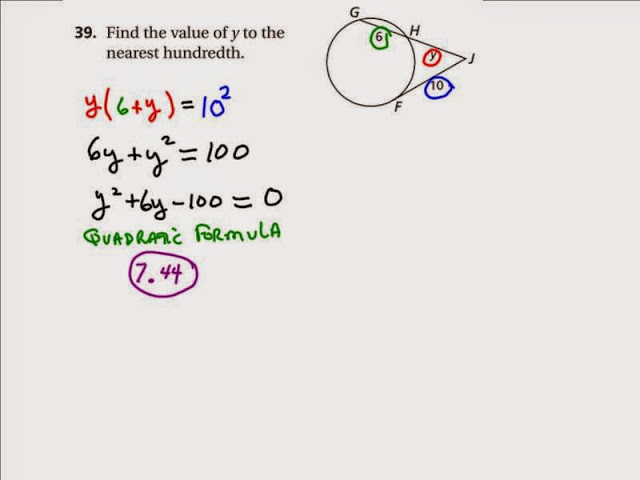
38, 39 and 40

Pretty much the SAME

******************************************************************

problems

CLICK HERE FOR THE YEAR END REVIEW

Click on an image to see a larger view

CONTENTS

INTRO to GEOMETRY - Points, Lines, and Planes

INTRO to GEOMETRY - Segments and Their Measures

INTRO to GEOMETRY - Angles and Their Measures

INTRO to GEOMETRY - Segment and Angle Bisectors

INTRO to GEOMETRY - Angle Pair Relationships

INTRO to GEOMETRY - Perimeter, Circumference, and Area

INTRO to GEOMETRY - Misc.

PROOF - Inductive Reasoning

PROOF - Conditional Statements

PROOF - Deductive Reasoning

PROOF - from Algebra

PROOF - about Segments

PROOF - about Angles

PROOF - Perpendicular Lines

PROOF - Parallel Lines and Transversals

PROOF - in the Coordinate Plane

PROOF - Misc.

CONGRUENT - Triangles and Angles

CONGRUENT - Proving using SSS and SAS

CONGRUENT - ASA and AAS

CONGRUENT - using CPCTC

CONGRUENT - Isosceles, Equilateral, Right Tri

CONGRUENT - Coordinate Proof

CONGRUENT - Misc.

TRIANGLES - Perpendiculars and Bisectors

TRIANGLES - Medians and Altitudes of a Triangle

TRIANGLES - Inequalities in One Triangle

TRIANGLES - Misc.

POLYGONS - Definitions and types

POLYGONS - Quadrilaterals

POLYGONS - Properties of Parallelograms

POLYGONS - Proving Quads are Parallelograms

POLYGONS - Proving Quads are Rhombuses

POLYGONS - Proving Quads are Rectangles or Squares

POLYGONS - Properties of Trapezoids and Kites

POLYGONS - Misc.

SIMILAR - Ratio and Proportion

SIMILAR - Proportions in Geometry

SIMILAR - Similar Polygons

SIMILAR - Proving Triangles are Similar

SIMILAR - Misc.

RIGHT TRIANGLES. - The Pythagorean Theorem

RIGHT TRIANGLES. - Converse of the Pyth.

RIGHT TRIANGLES. - Special Right Triangles

RIGHT TRIANGLES. - Misc.

TRIG - Right Triangles and Trig

TRIG - Similar Right Triangles

TRIG - Trigonometric Ratios

TRIG - Solving Right Triangles

TRIG - Vectors

TRIG - Misc.

CIRCLES - Tangents

CIRCLES - Segment Lengths

CIRCLES - Arcs and Chords

CIRCLES - Inscribed Angles

CIRCLES - Other Angles

CIRCLES - Equations of Circles

CIRCLES - Misc.

DISTANCE - two points in plane

DISTANCE - Perimeters of Similar Figures

DISTANCE - Circumference and Arc Length

DISTANCE - Misc.

AREA - Circles

AREA - Regular Polygons

AREA - Similar Figures

AREA - Circumference and Arc Length

AREA - Areas of Circles and Sectors

AREA - Misc.

TRANSFORMATIONS - Motion in a Plane

TRANSFORMATIONS - Rotations

TRANSFORMATIONS - Reflection

TRANSFORMATIONS - Translations and Vectors

TRANSFORMATIONS - Misc.

SOLIDS - Surface Area and Volume in General

SOLIDS - Surface Area of Prisms and Cylinders

SOLIDS - Surface Area of Pyramids and Cones

SOLIDS - Surface Area of Spheres

SOLIDS - Volume of Prisms and Cylinders

SOLIDS - Volume of Pyramids and Cones

SOLIDS - Volume of Spheres

SOLIDS - Misc.

INTRO to GEOMETRY - Points, Lines, and Planes

Point

A specific place in space. They are infinitely small (they have no size)

and are denoted by a single capital letter,

in this case, A.

Line

A straight path that extends infinitely in both directions.

Lines are denoted by two points on the line under a line symbol, <->.

 Also, they are made up of an infinite number of points.

Plane

A flat surface that extends infinitely in all directions.

Planes have no thickness whatsoever and can be made using a minimum of three points.

 Shown here is a plane being intersected by two lines.

Planes are named by four points on the plane

(i.e. "plane ABCD").